.: Is Graham Priest's Dialetheism a Logic of Quantum Mechanics?

Thursday, 27 October 2016

Is Graham Priest's Dialetheism a Logic of Quantum Mechanics?

Graham Priest tells us that in certain quantum-mechanical experiments “the law of excluded middle tells us that [an atom, particle, etc.] is one or the other”. He also claims that quantum mechanics (or at least its interpretations) suggests otherwise.

It’s tempting to think that the nature of quantum mechanics is the primary reason why the philosopher and logician Graham Priest (1948-) defends, accepts and uses dialetheic logic (or dialetheism generally).¹ Indeed, he mentions quantum mechanics (QM) a few times in various papers and in interviews.

It can now be asked if his views have any impact on the “classical world” or macro-reality (or, at the least, the world as it’s perceived and/or experienced). Perhaps, if dialetheic logic (DL) is truly dependent on the findings of quantum mechanics, it can also be asked if dialetheism is applicable to the “classical world” at all. Of course I may be barking up the wrong tree here. That is, why assume that this macro-world/micro-world opposition has any relevance to dialetheic logic?²

In any case, what has Priest got to say about what he calls “[u]nobservable realms”? (A term which needn’t only refer to the quantum world; but also to historical events, events beyond our galaxy, numbers/abstract objects, etc.) Take this passage from Priest:

“[I]t would sometimes (in the well-known two-slit experiment) appear to be the case that particles behave in contradictory fashion, going through two distinct slits simultaneously.”

Dialetheic logic would be an ideal logic to describe (or capture) such a phenomenon. Nonetheless, Priest’s clause “it would sometimes… appear” does seem to qualify things a little. In other words, since Albert Einstein, hasn’t the supposed “contradictory” (or “paradoxical”) nature of quantum phenomena — or states — been questioned by various physicists (including David Bohm, many-worlds theorists, etc.)?

More broadly, Priest says that “inconsistent theories may have physical importance too”. At first blush, the following can now be asked:

Does inconsistency necessarily have a connection with dialetheism?

And, if so, what’s the connection in particular instances?

What Priest continues to say may mean that not only is the statement above unconnected to dialetheism; it may not be connected to quantum mechanics either. Priest himself continues:

“An inconsistent theory, if the inconsistencies are quarantined, may yet have accurate empirical consequences in some domain. That is, its predictions in some observable realm may be highly accurate.”

Since Priest is talking about an “observable realm”, this mustn’t be about quantum mechanics. (Then again, some of the clues as to the reality of the quantum world are indeed observable.) Not only that: talk of the “accurate empirical consequences” of suspect, questionable and even false theories is something that’s widely accepted throughout the sciences and in the philosophy of science. Indeed Priest finishes off by saying that “one may take the theory, though false, to be a significant approximation to the truth”.³

Priest’s Examples From Quantum Mechanics

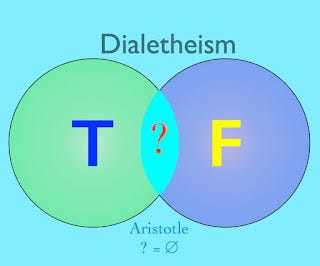
Here’s a passage from Priest on an aspect of quantum mechanics that’s relevant — or not! — to dialetheism:

“Unobservable realms, particularly the micro-realm, behave in a very strange way, events at one place instantaneously affecting events at others in remote locations.”

It’s difficult to see how this physical phenomenon has any direct relevance to dialetheism. Nothing contradictory (or paradoxical) is happening here; unlike the two-slit experiment. What’s does happen, however, does indeed go against common-sense views of causation and also against “classical” (or “local”) physics. Nonetheless, that doesn’t automatically entail contradiction or paradox.

Priest also gives the example of radioactive decay. He writes:

“[S]uppose that a radioactive atom instantaneously and spontaneously decays. At the instant of decay, is the atom integral or is it not?”

Now for the traditional logic of this situation. Priest continues:

“In both of these cases, and others like them, the law of excluded middle tells us that it is one or the other.”

Couldn’t the atom be neither integral nor non-integral when it instantaneously and spontaneously decays? (Priest talks of either/or or “one or the other”; not neither/nor.) Or, alternatively, at that point it may not be an atom at all!

This appears to be a temporal problem which must surely incorporate definitions — or philosophical accounts — of the concepts [instantaneously] and [spontaneously].⁴ Nonetheless, if they define time instants that don’t exist (the period from t¹ to t² doesn’t exist), then Priest and others may have a point. However, can an atom — or anything else — “decay” (or do anything) in a “timespan” which doesn’t actually exist? How can decay — or anything else for that matter — occur if there’s no time in which it can occur?…

So what about Priest’s own conclusion when it comes to atomic decay?

Priest claims that the aforementioned atom “at the point of decay is both integral and non-integral”. This isn’t allowed — Priest says — if the law of excluded middle is globally accurate or true. The law of excluded middle tells us that the said atom must either be integral or non-integral; not “both integral and non-integral”.

Again, none of this (basically) philosophy of science — on Priest’s part — is solely applicable to quantum mechanics or dialetheism. The word “solely” was just used because Priest does indeed give another example from QM. He states that

“those who worked on early quantum mechanical models of the atom regarded the Bohr theory [as] certainly inconsistent”.

And “yet its empirical predictions were spectacularly successful”.

Needless to say, it must be stressed here that the word “inconsistent” is very different to the word “contradictory” (or “paradoxical”). Something can indeed be inconsistent because contradictory. However, can’t something also be inconsistent without being (logically) contradictory?

Abstract objects were mentioned in brackets earlier and Priest himself “move[s] away from the empirical realms” to “the realm of sets”.

Dialethic Logic and the Paradoxes of Set Theory

Priest claims that this realm “appears to be inconsistent” too.

Here again Priest uses the word “appears”. There are indeed paradoxes in set theory. However, haven’t logicians and mathematicians — like quantum physicists — attempted to rectify those inconsistencies or paradoxes? True, unsolved, these inconsistencies or paradoxes (again like QM) are perfect specimens to be dealt with — and captured by — dialethic logic. Nonetheless, it can be said that what’s captured isn’t a world (or reality) of any kind: it’s simply an unsolved paradox or inconsistency. In addition, just as it was asked earlier about how dialethic logic could be applied to the empirical world, so it can now be asked what the connection is between the paradoxes of set theory and the empirical world. That is, it can be argued that set theory needn’t have a necessary connection to such a world. (This will depend on a whole host of factors; such as what one’s take is on the reality — or existence — of numbers, sets, the members of sets, etc.)

If one believes in abstract objects such as sets, then they must exist in an abstract world. Thus dialethic logic, in this case at least, may be applicable to a world — an abstract world. (These vaguely Platonist announcements about sets and abstract objects will, of course, be rejected by certain philosophers, logicians and mathematicians.) ⁵

Conclusion

If dialetheic logic is all about quantum mechanics, then why not call it a logic of quantum mechanics? This means that if dialetheic logic is (as it were) justified by the nature of quantum reality, then it must also depend on that reality.

That said, there’s a tradition in the philosophy of logic which states that logic doesn’t depend on anything — least of all on the nature of the (or a) world. (Ludwig Wittgenstein, at one point, stated this position; and the early Bertrand Russell took the contrary view.) If that were the case, then it may also be the case that dialetheic logic isn’t dependent on the nature of quantum mechanics. It just so happens that QM sometimes — or many times — behaves in a way which can be captured by dialetheic logic. Yet that doesn’t also mean that dialethic logic is dependent on QM. And it doesn’t mean that dialethic logic is derived — in any way — from QM either.

In any case, Priest does clarify his position by arguing that

“the micro-realm is so different from the macro-realm that there is no reason to suppose that what holds of the second will hold of the first”.

True. However and again, is dialetheism independent of quantum mechanics? What’s more, is dialethism applicable to the “classical world”?

As already hinted at, perhaps Priest would reply:

What does it mean to ask if dialethic logic is applicable to the classical world?

Indeed need logic be applicable to the (or even a) world at all?

*****************************

Notes

(1) This case parallels — at least to some extent — ontic structural realism, which (it can be argued) is similarly motivated by the reality of quantum mechanics. Or to put that another way: what relevance does much of the ontic-structural-realist position have to the macro/classical world? (See here for my discussion of this subject.)

(2) Graham Priest is also inspired by Buddhist logic (or simply by Buddhist thought — see here). So, conceivably, this piece may just as justifiably have been entitled ‘Is Graham Priest’s Dialetheism a Logic of Buddhism?’.

(3) This chimes in with Karl Popper’s verisimilitude in which scientific theories have quantifiable truth-to-falsity contents.

(4) Perhaps, as hinted at earlier, what’s needed is some good old-fashioned conceptual analysis of the words “integral”, “non-integral”, “decay”, “spontaneously” and “instantaneously”. Not, of course, the kind of conceptual analysis which historically disappeared up its own backside (i.e., by ignoring science completely). Nonetheless, if quantum reality is the way many physicists say it is, then much conceptual analysis on this matter will either be wrong or simply inapplicable to the quantum domain.

(5) What is — or what constitutes — a world anyway?